Class content > Energy: The Quantity of Motion  

Prerequisites

• Kinetic energy and the Work-Energy Theorem
• Energy of place -- potential energy
• The gradient -- a vector derivative

From our analysis of the work-energy theorem and conservative forces, we learned that we could express the work done by certain forces (gravity, electricity, and springs, for example) in terms of a simple function of position (separations of the interacting objects) -- the potential energy. In many situations, this is a much more convenient quantity to work with than forces since it is a scalar -- it has no direction. Forces always have to be added vectorially with all the complexity that entails. To add potential energies, you just add them, of course being careful of the signs!

Despite the fact that the potential energy is a scalar without a direction associated with it, nonetheless, it still contains the vector information about the direction of forces. The way it does this is by how it changes in space -- its derivatives. Recall that the basic definition of the potential is the negative of the work done by a conservative force along the direction of motion:

If we reverse this, solving for the force, we get:

The force in the direction of the displacement is the derivative of the potential energy in that direction. We use the marker "type" to indicate that the kind of force we get comes from the kind of PE we start with. Electric PE gives the electric force, gravitational PE gives the gravitational force, etc.

If our displacement was in the x direction, we would get the more straightforward derivative:

and we would get the x-component of the corresponding force. We would get analogous results if we took our displacements in the y or z directions. We can combine these to get the full vector of the force corresponding to the potential energy:

This particular combination of the spatial derivatives gives the full vector of the force. The upside-down vector delta on the right just is a shorthand for the stuff in parentheses. It is called the gradient and is useful in talking about how a function changes in space. (We use the curly or "partial" dees, "∂", to remind ourselves that U is a function of all three variables, (x, y, z), and when we take a derivative with respect to one of those variables, we hold the other variables fixed.)

Although this is using somewhat advanced math (vector calculus), all it is really saying is:

The force associated with a potential energy function points in the direction that the potential energy is falling the fastest.

Getting forces from PE in 1D

In 1D this is straightforward. The direction of the force is "down the hill". The figure below, (taken from Interpreting Mechanical Energy Graphs) shows the PE function for a mass attached to a light ("massless") spring whose other end is attached to the wall. The position x = 0 corresponds to the relaxed (unstretched) position of the spring.

The negative slope of the PE curve tells the direction and magnitude of the force the spring exerts on the object. When the object is at the bottom of the curve, where the PE curve is flat (at x = 0) there is no force. As the object moves to the right, the force points to the left (downhill) and grows as the mass moves farther out, stretching the spring more. When the object is on the left (negative values of x) the force points to the right -- again downhill -- and grows in magnitude as you go further from the origin.

Getting forces from PE in 2D

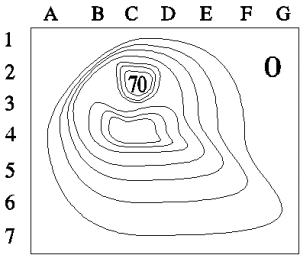
In 2D, it's a little trickier. Here, it's convenient for us to draw lines that correspond to constant values of the potential (equipotential lines). This is like a topographic map that shows the height above sea level. A schematic one is shown at the right. The "0" at the upper left marks sea level. Each line you cross moving toward the "70" corresponds to adding another 10 m of height. The map describes a broad hill with two small peaks at the top.   Lets consider the changes in PE and forces when you move along the lines of constant height.  Since you are staying at the same height, gravity does no work on you and walking is easy. Since the gradient points in the direction in which the height changes most, it is always perpendicular to the lines of constant height, pointing along the most rapid descent. There is a steep hill in the map at the right near B2 and the rise to the top of the peak marked "70" at the top of the graph is also quite steep and would be a hard climb.

Getting forces from PE in 3D

3D potential energies are of considerable importance in understanding electrical forces between atoms and molecules. But showing a 3D potential energy map is hard on a 2D surface like this computer screen. But you can get the idea from various 2D projections such as the one shown in the figure at the right. This is a slice of a 3D situation with two sheets of charge (one + ,one -) with a hole in it -- a model of a membrane with a pore. In this case, the "lines" of equal potential become surfaces and the force associated with the change in the potential points perpendicular to these surfaces. We will work on these issues more after we have introduced the concept of electric potential (the electric analog of gravity's "g") when we discuss electric fields.   In this example, inside the membrane, the electric force is strong and almost constant, pointing perpendicular to the closely packed sheets (shown here as lines) of equipotential surfaces. But outside the membrane, where the equipotential surfaces are far apart, the derivative of the potential is much smaller so the forces are less.

Joe Redish 11/24/12

Wolfgang Losert 11/26/12